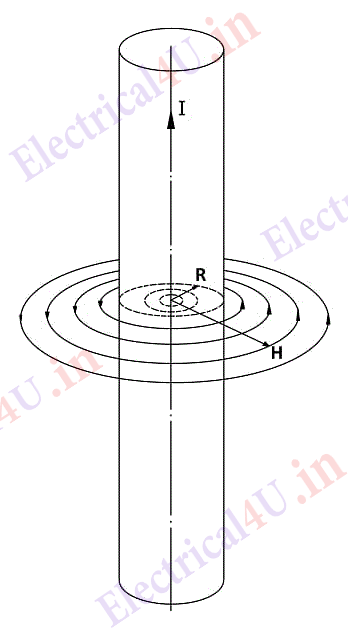
Figure 1 shows a straight solid conductor in which the steady current I is flowing in upwards direction. The magnetic flux is produced due to current flowing in the conductor. The magnetic flux is produced outside the conductor along its complete length. The flux lines are in one plane and are perpendicular to the direction of current. The arrows on the circular path shows the direction of the magnetic field produced. Just as the magnetic field is produced outside the conductor, magnetic field is also produced inside the conductor. Magnetic field intensity or magnetic field strength is denoted by H. The direction of the magnetic field intensity is shown in the figure at a point distant r from the center of the conductor.
Ampere’s circuital law states that the current I enclosed will be the integral around the closed path of the component of H along the path times the differential length (dL) of the path.
∮HdL = I enclosed …(equation1)
If θ is the angle between vectors H and dl at every point on the closed path,
∮HdL cos θ = I …(equation2)
Applying Ampere’s law to the case under consideration,
2π r H = I …(equation3)
i.e. the product of H and the distance around the closed path is equal to current enclosed from (equation3)
H = I/2π r A per meter …(equation4)
Where I is in Ampere and r in meters.
The same principle can be used for finding the value of H at any point inside the conductor. Thus if the distance r < R then applying Ampere’s law, the current enclosed in the conductor upto distance r,
Ir = (πr2/πR2) I …(equation5)
Where I is the total current carried by the conductor. This is on the assumption that the total current is uniformly distributed over the whole cross section of the conductor πR2
From (equation5) 2πrHr = (r2/R2)I
Or
Hr = (Ir/2π R2) amps/meter …(equation6)
This relation is applicable when r<R.
